Introduction

This is a progress report on the muon identifier simulations studying various Level 1 trigger road rates and the effect of charge particle detection efficiency on the performance.

Trigger Roads

This note addresses the PHENIX muon identifier LVL-1 trigger performance for the pad geometry and trigger road scheme discussed in Chapter 11 of the PHENIX CDR-Update. Other readout topologies, channel counts, and trigger algorithms are under study but not discussed here.

A sample of 500 "UA1 events" was produced using PISA and analyzed using PISORP. These results concerning trigger road finding are based mostly on a modified version of the GSU road finding algorithm (mun_l1_trg) now called mun_lv1_trg. (The modified version stores additional information and is no longer restricted to a maximum of 5 trigger roads.)

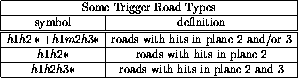
The trigger roads used here are defined and described in the CDR-Update. The analysis counts the number of events which have at least a specified minimum number of roads (1 or 2) satsifying a particular depth requirement. All roads are required to have a hit in muon identifier plane 1. For this particular note, hits in layers 4-6 play no role. The following three types of road depths are considered.

Assuming a perfect charged particle detection efficiency, the row labelled "all charged hits" of Table 1 shows the number of events with two or more roads each of which has at least the indicated depth. For instance, 51 out of 500 events have two or more roads, each of which has hits in layers 1, 2, and 3 (and perhaps subsequent layers). This indicates that with no real vector meson decays present (and neglecting beam gas events), the apparent dimuon trigger rate would be approximately 10% of the Au-Au central collision rate if roads are required to have hits in each of the first three planes. Also, Table 1 shows that 104 events have two or more roads extending to at least layer 2. This corresponds to a 20% apparent dimuon trigger rate for a trigger which only requires hits in the first two layers. Finally, this row of Table 1 shows that 109 events have two or more roads each of which has a hit in layer 2 and/or layer 3.

The next row of Table 1 labelled "muon hits" shows the number of events for each of the cases described above which are due to real muons. These muons come from pion decay and are an irreducible background. The contribution due to real non-muons (ie, pions) are listed in the row labelled "non-muon hits." Finally, the row labelled "accidentals" gives the number of triggers due to fake roads created by unrelated hits from multiple tracks. Note that the values are a small fraction of the total.

The corresponding single muon rates (for which just 1 or more road of the appropriate type is required) are given in the second half of Table 1.

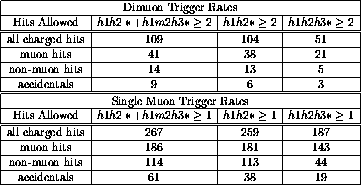
Table 1: Number of apparent dimuon and single muon triggers for 500 UA1 events for the CDR-Update trigger scheme and pad geometry and for a 100% charged particle detection efficiency in all planes.

We repeated the analysis used to create Table 1 but changed the simulated charged particle detection efficiencies to 98% for all planes. The results are shown in Table 2. Results for 95% and 90% charged particle detection efficiencies are shown in Tables 3 and 4, respectively.

Table 2: Number of apparent dimuon and single muon triggers as described in Table 1 but with a 98% charged particle detection efficiency in all planes.

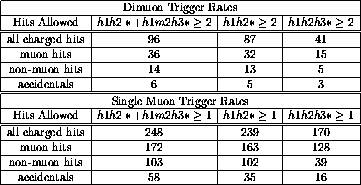
Table 3: Number of apparent dimuon and single muon triggers as described in Table 1 but with a 95% charged particle detection efficiency in all planes.

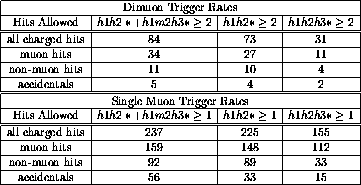
Table 4: Number of apparent dimuon and single muon triggers as described in Table 1 but with a 90% charged particle detection efficiency in all planes.

These numbers are all based on the GEISHA hadronic interaction component of GEANT. Studies using FLUKA are in progress and are expected to give higher rates based on preliminary results and also on Earl Cornell's thesis work.

Finally, to study the corresponding efficiencies of these triggers, we have studied 2380 simulated 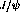decays into two muons. The results are show in Table 5. For each of the 2380 events, both muons were generated between 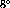and 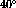of the beam line. A total of 1673 events have both muons reaching layer 1 of the muon identifier. A total of 2306 have at least one muon reaching layer 1.

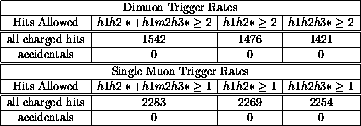
Table 5: Number of apparent dimuon and single muon triggers as described in Table 1 for 2380 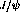decays into two muons with a 100% charged particle detection efficiency in all planes.